Gven
The lograthmic function, lograthmic fu
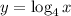
To find:
a) Rewrite the function in exponential form.
b) For each given value of y, find the value of x to complete the ordered pair.
c) The graph of the function.
Step-by-step explanation:
It is given that,
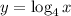
a) Since,
It is known that,
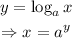
Then,

Hence, the exponential form of the function is,

b) Also,
For y = -2,.
The value of x is given by,

Hence, the ordered pair is (1/16, -2).
For y = -1,
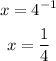
Hence, the ordered pair is (1/4, -1).
For y = 0,
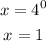
Hence, the ordered pair is (1, 0).
For y = 1,

Hence, the ordered pair is (4, 1).
For y = 2,
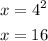
Hence, the ordered pair is (16, 2).
c) Also, the graph of the given function is,