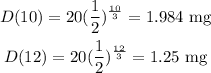Since the time of the half-life is 3 hours, then we have to divide the number of hours given by 3
The form of the exponential function is
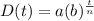
a is the initial amount
b is the factor of increasing or decreasing
In our situation:
Half-life means b = 1/2
Since the initial amount given is 20 mg, then
a = 20
Since the time of half-life is 3 hours, then
t must be the time divided by 3
The function which represents the situation is
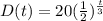
Since t = 3, then
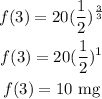
a.
At t = 6
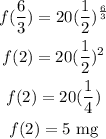
At t = 9
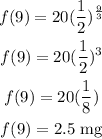
b.
To find the amount at t = 10 hours, substitute t by 10
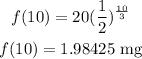
We know that by using the exponential function above
2.
The function form is
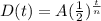
Where A = 20 -------- initial amount
n = 3 ------- the period of half-life
The formula is
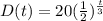
3.
The drug remains after 1 hour means substitute t by 1 first, then divide the answer by the initial amount, and change it to percent
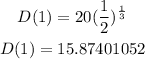
We will find the percent

To find the percent of the amount eliminated subtract 79.37% from 100%

4.
The direction for adults is
Do not exceed 4 doses per 24 hours
5.
Since the table has a period of 2 hours, then we will use t = 2, 4, 6, 8, 10, 12 in the formula above to find the amount of Dex.