The ending balance is 59,139.60
Here, we want to find the balance for a retirement account in which the initial deposit is compounded monthly at a certain percentage
Mathematically, we proceed to use the compound interest formula ;
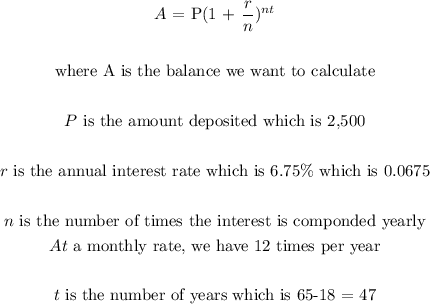
So, we proceed to insert these values;
