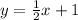Question

Write an equation perpendicular to y = -2x - 7 that passes through the point (4, 3).

Answer 1

We are asked to find the equation of a line that is perpendicular to the line y = -2x - 7 and passes through the point (4,3)

Recall that the standard form of the equation of a line in slope-intercept form is given by

`y=mx+b`

Where m is the slope and b is the y-intercept.

Comparing the standard form with the given equation we see that the slope is -2

`m=-2`

Since we are given that the lines are perpendicular so the slope of the other line must be negative reciprocal of the given line.

`m=(1)/(-(-2))=(1)/(2)`

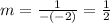
So the slope of the required equation is m = 1/2

Since we are also given that the line passes through the point (4,3)

The point-slope form of the equation of a line is given by

`y-y_1=m(x-x_1)`

Let us substitute the value of slope and the given point into the above equation.

`y-3_{}=(1)/(2)(x-4_{})`

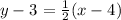
Solving the equation for y.

`\begin{gathered} y-3=(1)/(2)x-(4)/(2) \\ y-3=(1)/(2)x-2 \\ y=(1)/(2)x-2+3 \\ y=(1)/(2)x+1 \end{gathered}`

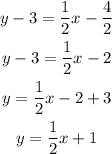
Therefore, the equation of the line is

`y=(1)/(2)x+1`