Account A:
Initial Deposit 1000
2.42% for 4 years
Account B:
Initial Deposit 1000
2.42% in 24 months
We have to find the simple interest earned in each account. The formula for simple interest is:
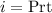
Where
i is the interest earned
P is the initial amount
r is the rate of interest, in decimal
t is the tiem
For account A:
P = 1000
r = 2.42% = 0.0242
t = 4 years
Thus, the interest earned is:
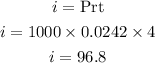
For account B:
P = 1000
r = 0.0242
t = 24 months = 24/12 = 2 years
Thus, the interest earned is:

example:
i = Prt
Since we want an example where interest amount is same in a a year, we know i and t are constant. We just need to figure out two P's and two r's that give the same value.
Suppose the initial deposit is 2000 and the rate of interest is 5%.
Now, suppose intial deposit is 500 and rate of interest is 20%.
They would both equate the same interest in 1 year.