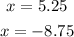1 Answer

Nadeem Ahmad · Answer 1 · 2023-02-16T17:07:30+0000

To solve this equation, we first need to solve for the absolute value part:

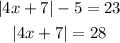
$\begin{gathered} |4x+7|-5=23 \\ |4x+7|=28 \end{gathered}$

Now, we need to consider two possibilities:

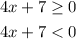
$\begin{gathered} 4x+7\ge0 \\ 4x+7<0 \end{gathered}$

In the first case, the absolute value will return the same as inside the vertical bars:

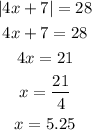
$\begin{gathered} |4x+7|=28 \\ 4x+7=28 \\ 4x=21 \\ x=(21)/(4) \\ x=5.25 \end{gathered}$

In the second case, the absolute value will give the same but with opposite sign:

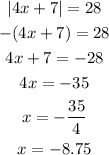
$\begin{gathered} |4x+7|=28 \\ -(4x+7)=28 \\ 4x+7=-28 \\ 4x=-35 \\ x=-(35)/(4) \\ x=-8.75 \end{gathered}$

So, the solutions are:

$\begin{gathered} x=5.25 \\ x=-8.75 \end{gathered}$

Please log in or register to add a comment.