Given the equation:
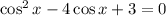
Let's find all the solutions of the equation in the interval: [0, 2π).
To find the solution, take the following steps.
Step 1:
Let u = cos x.
Then factor the left side of the equation.
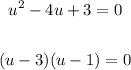
Step 2:
Now, replace u with cosx:
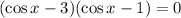
Step 3.
Equate the individual factors to zero and solve for x:
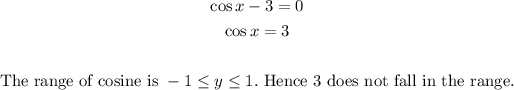
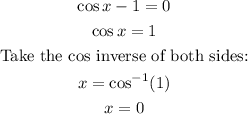
This cosine function is positive in the first and fourth quadrants.
To find the second solution, subtract the reference angle from 2π to get the second solution in quadrant IV.
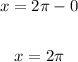
Therefore, the solution in the given interval is:
x = 0
ANSWER:
x = 0