The correct option is
D. (x-4)^2 + (y-2)^2 = 9
The equation of a circle that has center in a point P = (h, k) is:
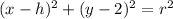
Where r is the radius. In this case the center is in (4, 2). Then we can rplace h and k:
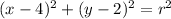
All that is left is replace r by the radius. In this case r = 3 then r^2 = 9
Now we can complete the equation:
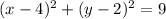
And that's option D.