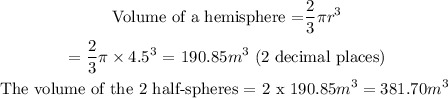Question

find the surface area of the part of the cylinder that is outside of the figure. find the surface area of the wmitre outside of the satellite. find the volume of the 2 half spheres.

Answer 1

PART 1 -To find the surface area of the part of the cylinder outside the figure.

The part of the cylinder outside the figure is a hemisphere (both sides)

`\begin{gathered} \text{Area of a hemisphere }(\text{curved surface only) }=2\pi r^2 \\ \text{Radius of the hemispher = }\frac{\text{Diameter}}{2}=(9)/(2)\text{ = 4.5m} \\ Curved\text{ surface area of a hemisphere = 2}\pi\text{ }*4.5^2\text{ = }127.23m^2\text{ (2 d.p)} \\ For\text{ the 2 hemispheres, } \\ \text{Curved surface area = 2 x 127.23 = 254.46m}^2 \end{gathered}`

PART 2 - To find the surface area of the entire figure:

`\begin{gathered} \text{Total Area =Curved Surface Area of the Cylinder+Hemispheres} \\ \text{Curved Surface Area of the Cylinder = 2}\pi rL \\ =\text{ 2}\pi\text{ }*4.5*\text{ }19\text{ } \\ =537.21m^2\text{ (2 decimal places)} \\ \text{Therefore, Total Area = 537.21 + }254.46 \\ =\text{ 791.67}m^2\text{ } \end{gathered}`

PART 3 - To find the volume of the two half-spheres (hemisphere)

`\begin{gathered} \text{Volume of a hemisphere =}(2)/(3)\pi r^3 \\ =(2)/(3)\pi*4.5^3\text{ = }190.85m^3\text{ (2 decimal places)} \\ \text{The volume of the 2 half-spheres = 2 x 190.85}m^3=381.70m^3\text{ } \end{gathered}`