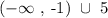Question

Graph each function. Then identify the domain and range. Only part 9 and 11. Skip part 10 and 12

Answer 1

Question 9 :

Given the piece-wise function:

`\begin{gathered} h(x)\text{ = }(4)/(3)x-2\text{ if x<0} \\ h(x)\text{ = -x + 1 if x }\ge\text{ 0} \end{gathered}`

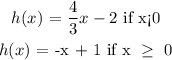
First, we graph the function as shown below:

The domain is the set of allowed inputs or values in the x-axis:

Domain:

`(-\infty,\text{ }\infty)`

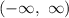
The range is the set of allowed outputs or values in the y-axis.

Range:

`(-\infty,\text{ 1\rbrack}`

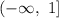
Question 11:

Given the piece-wise function:

`\begin{gathered} k(x)\text{ =x + 4 if x }<\text{ -1} \\ k(x)\text{ = }5\text{ if -1 }<\text{ x }<\text{ 2} \\ k(x)\text{ = }-(1)/(2)x\text{ + 1 if x}\ge\text{ 2} \end{gathered}`

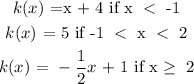
The graph of the function is shown below:

The domain: when x= -1, the function is undefined

`(-\infty,\text{ -1) }\cup\text{ (-1 , }\infty)`

The range: At x=-1, the function is undefined.

`(-\infty\text{ , -1) }\cup\text{ 5}`