Question

Uyen measures two rectangular tabletops. The green tabletop has dimensions of 7 feet by 4 1/2feet. The area of the brown tabletop is 1 1/2 times as great as the area of the green tabletop. What is the area of the brown tabletop?

Answer 1

We have that the area of the brown tabletop is given by the following expression:

`A_b=1(1)/(2)\cdot A_g`

Let's find first the area of the green tabletop. If the base measures 7 feet and the height is 4 1/2 feet, then:

`\begin{gathered} A_g=b\cdot h_{} \\ \Rightarrow A_g=(7)\cdot(4(1)/(2))=7\cdot((9)/(2))=(63)/(2)=31(1)/(2) \\ A_g=31(1)/(2)ft^2 \end{gathered}`

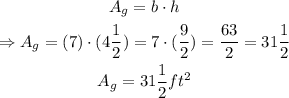
now that we know the area of the green tabletop, we can find the area of the brown tabletop:

`\begin{gathered} A_b=1(1)/(2)\cdot A_g \\ A_g=31(1)/(2) \\ \Rightarrow A_b=1(1)/(2)\cdot(31(1)/(2))=((3)/(2))((63)/(2))=(189)/(4)=47(1)/(4) \\ A_b=47(1)/(4)ft^2 \end{gathered}`

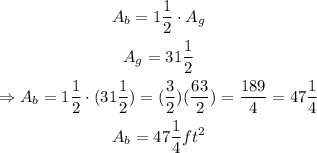
therefore, the area of the brown tabletop is 47 1/4 ft^2