Question

|5x-8|+5=32 how would i set this problem up to solve

Answer 1

You have the following expression:

`\lvert5x-8\rvert+5=32`

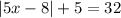
subtract 5 both sides of the equation:

`\begin{gathered} \lvert5x-8\rvert=32-5 \\ \lvert5x-8\rvert=27 \end{gathered}`

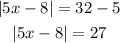
Take into account that the absolute value of any number is equal to the same number but positive.

Then, you have:

`5x-8=-27`

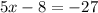
and

`5x-8=27`

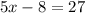
Then, solve the previous equations:

5x - 8 = -27 add 8 both sides

5x = -27 + 8

5x = -19 divide by 5

x = -19/5

5x - 8 = 27 add 8 both sides

5x = 27 + 8

5x = 32 divide by 5

x = 32/5

Then, the solutions of the given equations are:

x = -19/5

x = 32/5