Given:
The coordinates of point P(-4,0).
The coordinates of point A(2,-6).
Step-by-step explanation:
The distance of AP is 3/4 times of AE. So,
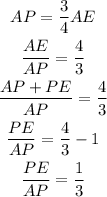
The formula for the coordinates of the point lying between endpoint (x_1,y_1) and (x_2,y_2) such that divide the line in m:n is,
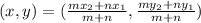
So coordinates for point P lying on segment AE is,

Solve the equations for x and y.

For y,
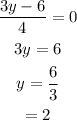
So coordinates of point E is (-6,2).