Given:
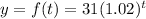
A) We are to write the exponential function in the form

When t = 1
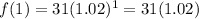
Also,
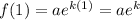
Equating the two equations and solving for k
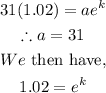
Apply exponent rules:
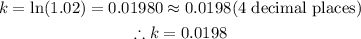
B) The annual growth rate is,
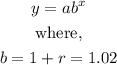
Equating the two expressions together and solving for r

Hence, the annual growth rate is 2% per year.
C) The continuous growth rate is the constant k which is

Hence, the continuous growth rate is 1.98% per year.