Question

Complete parts (a) through (d).a. Graph the function f(x)=1981+4e−2x for x=0 to x=10. b. Find f(0) and f(10).c. Is this function increasing or decreasing?d. What is the limiting value of this function?

Answer 1

a.

b.

`\begin{gathered} f\mleft(0\mright)=(198)/(1+4e^(-2\cdot0))=(198)/(1+4)=(198)/(5)=39.6 \\ f(10)=(198)/(1+4e^(-2\cdot10))=197.99\cong198 \end{gathered}`

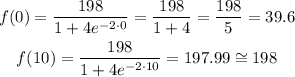
c. increasing

d. 198

Explanation:

We have the following function:

`f\mleft(x\mright)=(198)/(1+4e^(-2x))`

a.

From the function we can see that the factor with e (euler) when x increases will become smaller, therefore the values of the function will increase, the only graph that meets these characteristics is the following:

b.

We must calculate when x = 0 and when x = 10, like this:

`\begin{gathered} f\mleft(0\mright)=(198)/(1+4e^(-2\cdot0))=(198)/(1+4)=(198)/(5)=39.6 \\ f(10)=(198)/(1+4e^(-2\cdot10))=197.99\cong198 \end{gathered}`

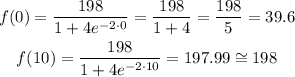
c.

The function is increasing since as the values of x increase, the values of y increase

d.

The limiting value for f (x) is 198, since the function will never be able to reach this value because there is a horizontal asymptote at that point