Question

For the function f(x)=2x² + 5x + 3, find the average rate of change of f from x=2 to x = 3.

Answer 1

We have a function f(x) for which we have to find the average rate of change from x = 2 to x = 3.

The function f(x) is defined as:f

`f(x)=2x^2+5x+3`

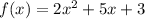
The average rate of change (r) for a function in a interval [a, b] can be expressed as:

`r=(f(b)-f(a))/(b-a)`

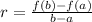
In this case, b = 3 and a = 2, we can calculate the average rate of change as:

`\begin{gathered} r=(f(3)-f(2))/(3-2) \\ \\ r=(2(3)^2+5(3)+3-(2(2)^2+5(2)+3))/(1) \\ \\ r=2(9)+15+3-2(4)-10-3 \\ r=18+15-8-10 \\ r=15 \end{gathered}`

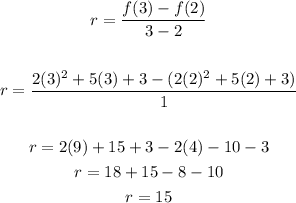
Answer: the average rate of change of f(x) from x = 2 to x =3 is 15.