The ideal gas equation is given as,
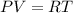
Here, P is the pressure, V is the volume, R is universal gas constant and T is the temperature.
Let consider the initial case, when pressure is P1, volume is V1 and temperature is T1. The ideal gas equation is given as,
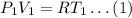
Consider the case, when pressure is P2, volume is V2 and temperature is T2. The ideal gas equatiojn is given as,
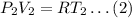
Dividing equation (1) by equation (2);
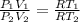
Therefore,
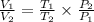
Since,

And,

Therefore,
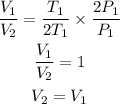
Therefore, the final volume V2 is equal to initial volume V1. Hence, option (b) is the correct choice.