Answer:
B and D are correct.
Explanation:
To find the area of a rectangle, simply multiply its length by its width. In this case, the length of the rectangle is
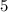 m and the width of the rectangle is
m and the width of the rectangle is
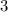 m, so its area must be
m, so its area must be
 square meters.
square meters.
To find the area of a triangle, divide the product of the triangle's base and height by
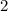 . As an algebraic expression, that would be
. As an algebraic expression, that would be
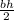 , where
, where
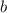 and
and
 are the triangle's base and height, respectively. In this case,
are the triangle's base and height, respectively. In this case,
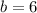 and
and
 , so the triangle's area is
, so the triangle's area is
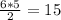 square meters.
square meters.
Now, let's look at the statements.
A: The triangle's area is
 square meters, not
square meters, not
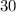 , so A is incorrect.
, so A is incorrect.
B: The rectangle's area is
 square meters and the triangle's area is also
square meters and the triangle's area is also
 square meters. Because the two figures have the same areas, their areas are equal, so B is correct.
square meters. Because the two figures have the same areas, their areas are equal, so B is correct.
C: We've already established that the two figures have equal areas, so one can't have a greater area than the other. Therefore, C is incorrect.
D: The rectangle's area is indeed
 square meters, so D is correct.
square meters, so D is correct.
Hope this helps!