We know that
• You have 2 1/2 yards of fabric.
,
• You want to make 3 pillows.
To find the number of yards of fabric you can use for each pillow, we just have to divide. We know that 2 1/2 is equivalent to 5/2 yards.
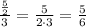
Therefore, you can use 5/6 yards of fabric for each pillow.