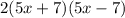Question

How can i solve this using difference of squares or sum or difference of cubes?50x^2 - 98

Answer 1

First, we can note that the greatest common factor of 50 and 98 is 2, so we can rewrite our expression as:

`2(25x^2-49)`

Now, the term into the parentheses is a difference of 2 squares because

`25x^2-49=(5x+7)(5x-7)`

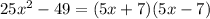
By substituting this result into the first expression from above, we get

`2(25x^2-49)=2(5x+7)(5x-7)`

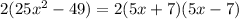
Therefore, the answer is:

`2(5x+7)(5x-7)`