To solve the exercise, it is helpful to draw the situation described in the statement:
Since we have the height after which the projectile will have to be at t seconds, then we replace h = 100 in the given equation and solve for t:
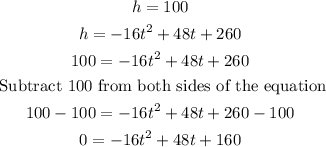
To solve the quadratic equation above, we can use the quadratic formula:
![\begin{gathered} x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}\Rightarrow\text{ Quadratic formula} \\ \text{For }ax^2+bx+c=0\Rightarrow\text{ Quadratic equation in standard form} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/52j3dlrg5nkusbfhfoos5ephvfrc40pqcw.png)
In this case, we have:
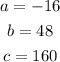
![\begin{gathered} x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \\ \text{ In this case, x = t} \\ t=\frac{-48\pm\sqrt[]{(48)^2-4(-16)(160)}}{2(-16)} \\ t=\frac{-48\pm\sqrt[]{2304+4(16)(160)}}{-32} \\ t=\frac{-48\pm\sqrt[]{2304+10240}}{-32} \\ t=(-48\pm112)/(-32) \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/l7uqcnaf1gl6vjffe5e3gtzjcuip5m10g8.png)
There are two possible solutions for the quadratic equation:
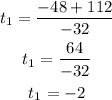
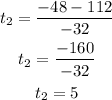
Since it makes no sense to say that time is negative, then the solution to the equation is t = 5.
Therefore, the projectile will be 100 feet above the canyon floor when 5 seconds have passed after its shot.