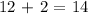In two years, Xavier will be 14 years old
Here, we want to get Xavier's age in 2 years
Let Xavier's current age be x and Madeleine's current age be m
The addition of the two will be 28
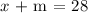
Secondly, we have that the age of Xavier x is 4 years older than half of Madeleine's age;
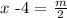
From the first equation, we can have a substition;

Now, put this in the second equation;

Xavier's current age is 12
In the next two years, his age will be;