Question

Hello! I need help with this question please make is easy to understand I am in year 9

Answer 1

When given an equation and then required to make one of the unknown variables the subject of the formula (equation), it simply means make the variable stand alone on one side of the equation while others stand on the other side. Right now, t is the subject of the formula, while the other variables are on the other side of the equality sign.

To make r the subject of the formula, we would have something which would look like;

`r=\ldots`

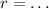
To begin, we would write out the equation;

`t=(r)/(r-3)`

Let us start by factoring out the letter r from the right side of the equation;

`(r)/(r-3)`

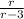
We cross multiply the equation by taking the following steps;

`\begin{gathered} t=(r)/(r-3) \\ \text{This can be re-written as;} \\ (t)/(1)=(r)/(r-3) \\ We\text{ cross multiply and this becomes;} \\ t*(r-3)=r*1 \end{gathered}`

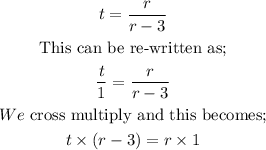
We can refine this to now become;

`rt-3t=r`

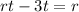
Next step we collect like terms. This means all terms that contains r would move to one side (either left or right), and all other terms would move to the other side. We now have the like terms as;

`rt-r=3t`

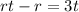
Note that the term r moves from the right to the left and becomes a negative (that is we now write rt - r). This is because any term that crosses the equality sign has its sign changed from positive to negative (or negative to positive).

As you can observe, -3t also crossed to the right side and became 3t.

Hence we can now factorize the left side as follows;

`\begin{gathered} rt-r=3t \\ r(t-1)=3t \end{gathered}`

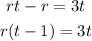
What we just did is;

`\begin{gathered} (rt)/(r)=t\text{ (r cancels out)} \\ \text{Also;} \\ -(r)/(r)=-1\text{ (r cancels out just like above)} \end{gathered}`

We now have the equation (formula) re written as shown above.

`r(t-1)=3t`

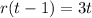
Note that the left hand side is actually a refined form of the following;

`r(t-1)\Rightarrow r*(t-1)`

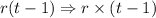
If we divide both sides by the other expression (t - 1), we can now make r to stand alone. This is shown as follows;

`\begin{gathered} \text{Divide both sides by} \\ (t-1) \\ (r(t-1))/((t-1))=(3t)/((t-1)) \end{gathered}`

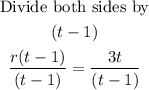
Notice that (t - 1) cancels out on the left side of the equation. We can now rewrite as;

ANSWER:

`r=(3t)/((t-1))`

We now have r as the subject of the formula.