Question

The functions g and h are given by g(x) = x + 1 and h(x) = x²

Find the values of x for which hg(x) = 3x² + x - 5

Answer 1

x = -
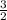`(3)/(2)` , x = 2

Explanation:

To find h(g(x)) substitute x = g(x) into h(x) , that is

h(g(x))

= h(x + 1)

= (x + 1)²

= x² + 2x + 1

For h(g(x)) = 3x² + x - 5 , then

3x² + x - 5 = x² + 2x + 1 ← subtract x² + 2x + 1 from both sides

2x² - x - 6 = 0 ← in standard form

(2x + 3)(x - 2) = 0 ← in factored form

Equate each factor to zero and solve for x

2x + 3 = 0 ⇒ 2x = - 3 ⇒ x = -
`(3)/(2)`

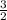
x - 2 = 0 ⇒ x = 2