Question

A lake with starts with 380 trout. Each year, the population decreases by 20. The population of trout in the lake after x years is represented by the function f(x)=380-20x.

Answer 1

To find the intercepts of a function we need to remember that:

• The x-intercepts happen when the value of the function is zero, then we equate the function to zero and solve for x.

,

• The y-intercepts happen when x=0, then we plug x=0 in the expression and the result is the intercept.

With this in mind we have that in this case the x intercept is:

`\begin{gathered} 380-20x=0 \\ 20x=380 \\ x=(380)/(20) \\ x=19 \end{gathered}`

Therefore the x-intercept is 19.

The y-intercept is:

`\begin{gathered} f(0)=380-20(0) \\ =380 \end{gathered}`

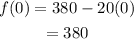
Therefore the y-intercept is 380.

Now we plot the intercepts and join them with a straignt line to get the graph:

The x-intercept means that it takes 19 years for there to be no trout in the lake. The y-intercept represents the number of trout that were in the lake at the beginning of the population decrease.