Since we have that y ≤ 2, the maximum value y can have is 2, so we have that:
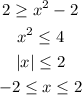
So the domain of the relation is D = [-2, 2].
The range is all y values the function can have.
Since the smallest value x² can have is 0, we have that:

So we have that y ≤ 2 and y ≥ -2, therefore the range of the relation is R = [-2, 2].