In general, the exponential growth/decay formula is
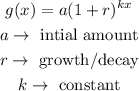
If 1+r>1, the function is growing while 1+r<1 implies that it is decaying.
In our case,
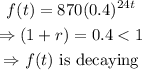
Furthermore,
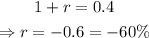
Thus, the function is decaying exponentially at a rate of 60%.
Finally, notice that t stands for the number of days; therefore, 24t implies that the function exponentially decays 60% a total of 24 times per day, and 24 times per day is equivalent to 1 time every hour.
Hence, the function decays 60% every hour.