Question

In planning her retirement, Liza deposits some money at 4.5% interest, with twice as much deposited at 5%. Find the amount deposited at each rate if the total annual interest income is $1450.She deposited $___at 4.5% and $__at 5%

Answer 1

we will call the amount deposited at 4.5% interest x.

Since she deposits twice as much at 5% interest. That means that if the amount deposited at 4.5% is x, the amount deposited at 5% is 2x.

• The interest income due to the money deposited at 4.5% interest is:

`\text{0}.045(x)`

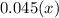
which is the 4.5% of the quantity x.

• The interest income due to the money deposited at 5% interes is:

`.05(2x)`

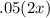
which is the 5% of the quantity 2x (the amount deposited at 5%).

Since the total annual interest income is $1450, the sum of the two previous expressions must be equal to $1450:

`0.045x+0.05(2x)=1450`

Solving the multiplication on the left side:

`0.045x+0.1x=1450`

combining the like terms on the left side:

`0.145x=1450`

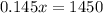
Dividing each side by 0.145

`(0.145x)/(0.145)=(1450)/(0.145)`

Making the divisions

`x=10,000`

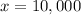
Answer:

The amount deposited at the 4.5% rate is $10,000, and

the amount deposited at 5% is twice as much: $20,000