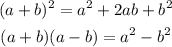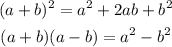
1) Let's expand the square of a binomial, using "a" for the first term and "b" for the second one:

The square of the first plus twice the product of them plus the square of b.
2) And the product of a sum and a difference:
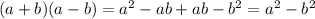
Note that the ab term was canceled out by -ab
The square of the first minus the square of the 2nd term
3) Hence, the patterns are: