Question

What is an equation of the line that passes through the point (6,1) and is

perpendicular to the line 2x + 3y 18?

Answer 1

y - 1 = 3/2(x - 6)

Explanation:

First, we need to find the slope of the given equation. Without a graph, the easiest way to do this is by isolating y.

2x + 3y = 18

3y = -2x + 18 (Subtract 2x from both sides)

y = -2/3x + 6 (Divide both sides by 3)

The slope is -2/3. Perpendicular lines have opposite reciprocal slopes, so a line perpendicular to this one would need to have a slope of 3/2. We're given a point for this line, so we can write the equation for it in point-slope form.

Point-Slope Form:
`y - y_(1)=m (x-x_(1))`

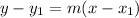
y - 1 = 3/2(x - 6)

If necessary, this can also be written in slope-intercept form by solving for y.