Number of observations = 201
SOLUTION
Problem Statement
The question tells us to find the total number of observations needed to be included in our sample given that we have a standard error of 0.28 using a 95% confidence interval with a variance of 4.1.
Method
In order to solve this problem, we need to follow the steps listed below:
1. We need to find the alpha level for the sampling. The formula for the alpha level is given by:

2. Find the critical probability (p*) from the alpha level gotten in step 1
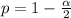
3. Convert the critical probability gotten above into a z-score (Z) by checking the z distribution table
4. Using the formula below, estimate the number of observations needed:
![\begin{gathered} \text{The initial formula is:} \\ ME=Z*\frac{\sigma}{\sqrt[]{n}} \\ \text{where,} \\ ME=\text{Margin of Error = Sampling distribution Error} \\ Z=\text{critical value gotten from z-table} \\ \sigma=\text{ standard deviation}=\text{square root of the variance} \\ n=\text{ number of observations} \\ \\ \text{Making n the subject of the formula, we have} \\ \therefore n=\mleft((Z*\sigma)/(ME)\mright)^2 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/mgxcnl62euc059up49bt4qwjws5s5dco01.png)
(Note: When the sampling is random, the maximum likely size of the sampling error is called the margin of error)
Implementation
1. We need to find the alpha level for the sampling:
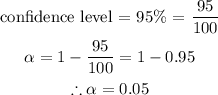
2. Find the critical probability (p*) from the alpha level gotten in step 1
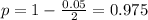
3. Convert the critical probability gotten above into a z-score (Z) by checking the z distribution table:
Notice that the two loops (red and blue) intersect at the critical probability value of 0.9750 on the z-table. We read off the Critical value (Z) from the vertical axis at the left of the page first then the horizontal axis at the top of the page.
Thus, the Critical Value (Z) = 1.96
4. Using the formula below, estimate the number of observations needed:
![\begin{gathered} n=((Z*\sigma)/(ME))^2 \\ \sigma^2=4.1 \\ \therefore\sigma=\sqrt[]{4.1} \\ \\ n=\mleft(\frac{1.96*\sqrt[]{4.1}}{0.28}\mright)^2 \\ \\ n=200.9\approx201 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/tu0nmdl2bp19nx8a7bux49xr4s8ao9nvzt.png)
Final Answer
The number of observations is: 201