To determine the probability of bull's eye, you have to determine the total possible area, which is the area of the square, and the area of the bull's eye, which is the area of the red circle.
Assuming that each square of the grid indicates one square unit, then each line indicates one unit, you can count the number of units that correspond to the side length of the square. I'll mark the line that crosses the center of the square as zero and use it as starting point to count:
The side length of the square is s=12 units, using the formula for the area you can calculate it as follows:

The radius of the red circle is r=1unit, using the formula for the area of the circle, you can calculate the area of the bull's eye as follows:
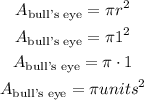
The probability of hitting the center of the target can be determined as follows:
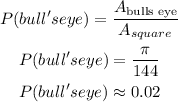
You can express the probability as a percentage by multiplying the result by 100:
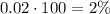
So, the probability of bull's eye is 2%