Let's find the first derivative of the function:

Let's find the critical point, in order to find its maximum:
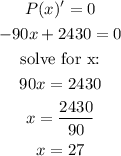
xo is a global maximum for the function if:
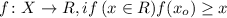
So:
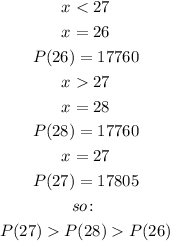
Therefore, x is the global maximum of the function, therefore, the automobile manufacturer needs to produce 27 cars per shift in order to maximize its profit