Question

The table and equation below each represent linear equations

Answer 1

Function 1 and function have the same rate of change.

The rate of change for the function 2 is the slope m = 5( The coefficient of x)

For the function 1:

`\begin{gathered} (x1,y1)=(0,5) \\ (x2,y2)=(5,20) \\ m=(20-5)/(5-0)=(15)/(5)=3 \end{gathered}`

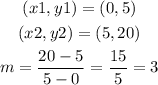
Since:

`5\\e3`

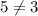
They dont have the same rate of change.

----------------------

The functions have the same value when x = 5.

For function 1:

`x=5,y=20`

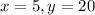
For function 2:

`\begin{gathered} y(5)=5(5)+20 \\ y=45,x=5 \end{gathered}`

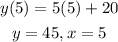
They have different values.

-----------------------------------

The sum of the rates of change for both functions is 8

`3+5=8`

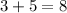
This is true