Question

Solve the equation f(x)=g(x) by graphing. f(x) = 2^x g(x) = 3/2x +1 How many solutions does the equation f(x)=g(x) have? Enter 0, 1, 2, or 3 in the box.

Answer 1

2 solutions (x = 0 and x = 2)

Step-by-step explanation:

The graph of the below functions is as shown below;

`\begin{gathered} f(x)=2^x \\ g(x)=(3)/(2x)+1 \end{gathered}`

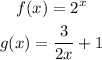
We can see from the graph that solutions of the equation f(x) = g(x) are x = 0 and x = 2. These are points of intersection of f(x) and g(x).

So there are two solutions of the equation f(x) = g(x).