Given the point:
(x, y) ==> (0, 0)
Number of hours the oil spilled for = 10 hours
Rate of increase = 2 inches per hour.
Let's find the equation that models the range of the spill's effect.
Apply the formula:

Where:
(h, k) is the center ==> (0, 0)
r is the radius
Since the oil spills for 10 hours and increases at the rate of 2 inches per hour, therefore, the radius of the circle will be:
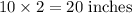
Therefore, the equation of the circle is:
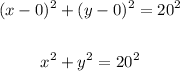
ANSWER:
