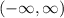The functions g(x) and h(x) are:
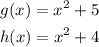
To find the composition (g°h)(x) we use the definition for the composition of functions:
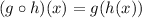
This means that to find the composition, we need to plug in h(x) as the value of x in g(x):
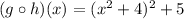
The next step is to simplify the expression. We use the formula for the binomial squared:
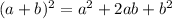
Applying this rule to the first term of the composite function:
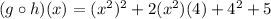
Simplifying the expression further:
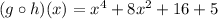
Finally, we combine 16+5 and we get 21 at the end of the expression:
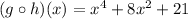
The composition of the functions is:
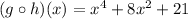
We also need to find the domain. The domain are the values allowed or possible for the x variable, in this case, we can have any value as the x value without problems. Any value is possible for x. Thus, the Domain is:
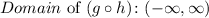
Answer:
Composition of the functions:
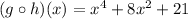
Domain: