Question

Determine the equation from the description.a) Given the graph f(x)=Ix-2I+3, what would be the equation of this same graph if it was shifted left one unit and down two units?

Answer 1

Give the equation of the graph:

`\text{ f(x) = }\lvert x-2\rvert\text{ + 3}`

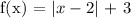
If it's been shifted by one unit to the left and two units downward, it means that the x-intercept will be subtracted by one unit and its y-intercept will be subtracted by two units.

Let,

g(x) = the new equation after being shifted

We get,

`\text{ f(x) = }\lvert x-2\rvert\text{ + 3 }\rightarrow g(x)\text{ = f(x-1) - 2}`
`g(x)\text{ = }\lvert(x-1)-2\rvert\text{ + 3 - 2}`
`\text{ g(x) = }\lvert x-3\rvert\text{ + 1}`

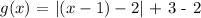
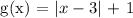
Therefore, the new equation of the graph after being shifted will be g(x) = Ix-3I + 1.