Question

A 2250 kg car traveling to the west slows down uniformly from 20.0 m/s to 5.00 m/s. How long does it take the car to decelerate if the force on the car is 8450 N to the east? How far does the car travel during the deceleration?

Answer 1

Given data

*The given mass of the car is m = 2250 kg

*The initial speed of the car is u = 5.00 m/s

*The given final speed of the car is v = 20.0 m/s

*The force on the car is F = 8450 N

The formula for the time taken by the car to decelerate is given as

`\begin{gathered} F=ma \\ =m*((v-u)/(t)) \end{gathered}`

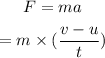
*Here a is the acceleration of the car

Substitute the known values in the above expression as

`\begin{gathered} 8450=2250*((20.0-5.00)/(t)) \\ t=4.0\text{ s} \end{gathered}`

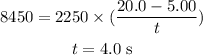
Hence, the time taken by the car to decelerate is t = 4.0 s

The formula for the distance travel by the car during the deceleration is given by the kinematic equation of motion as

`\begin{gathered} d=ut+(1)/(2)at^2 \\ =ut+(1)/(2)((v-u)/(t))t^2 \end{gathered}`

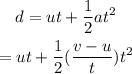
Substitute the known values in the above expression as

`\begin{gathered} d=(5.0)(4.0)+(1)/(2)((20.0-5.0)/(4.0))(4.0)^2 \\ =50.0\text{ m} \end{gathered}`

Hence, the distance travel by the car during the deceleration is d = 50.0 m