Answer:
C. 12m
Explanation:
Let the length of the shorter arm = x
Since the length of the longer arm is equal to twice the length of the shorter arm;
• The length of the longer arm = 2x
• The area of triangle ABD = 18√3 m².
Given a triangle with two sides and the included angle, the area of the triangle is calculated using the formula below:
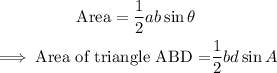
Therefore:
![18\sqrt[]{3}=(1)/(2)(x)(2x)\sin (60\degree)](https://img.qammunity.org/2023/formulas/mathematics/college/alber36x2nyh1t3757oyy2h0y40uzoi2r4.png)
We solve for x.
![\begin{gathered} 18\sqrt[]{3}=(2x^2)/(2)*\sin 60\degree \\ 18\sqrt[]{3}=x^2*\frac{\sqrt[]{3}}{2} \\ \text{Multiply both sides by }\frac{2}{\sqrt[]{3}} \\ 18\sqrt[]{3}*\frac{2}{\sqrt[]{3}}=x^2*\frac{\sqrt[]{3}}{2}*\frac{2}{\sqrt[]{3}} \\ x^2=36 \\ x^2=6^2 \\ x=6 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/7w2t4heol037u0oog3a2djd1hhle5cgrj5.png)
Multiply x by 2 to get the length of the longer arm:

The length of the longer arm is 12m.
C is the correct option.