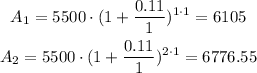Question

Suppose Juan borrows $5500 at an interest rate of 11 % compounded each year.Assume that no payments are made on the loan.Follow the instructions below. Do not do any rounding.(a) Find the amount owed at the end of 1 year$0(b) Find the amount owed at the end of 2 years.X5?

Answer 1

1 year: $6105

2 year: $6776.55

Explanation:

The formula for annual compound interest, including principal sum, is:

`A=P\cdot\mleft(1+(r)/(n)\mright)^(nt)`

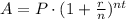
A = the future value of the investment/loan, including interest

P = the principal investment amount (the initial deposit or loan amount) = $5500

r = the annual interest rate (decimal) = 11% = 11/100 = 0.11

n = the number of times that interest is compounded per year = 1

t = the number of years the money is invested or borrowed for = 1 and 2

We replace in each case and we get the following:

`\begin{gathered} A_1=5500\cdot\mleft(1+(0.11)/(1)\mright)^(1\cdot1)=6105 \\ A_2=5500\cdot\mleft(1+(0.11)/(1)\mright)^(2\cdot1)=6776.55 \end{gathered}`