Question

How do you find the length of the longest side of a triangle

Answer 1

We will find the length of the longest side as follows:

*First: We can see that the other angle that is missing is 60°. Now, using the law of sines we find the other side of the triangle [Not the longest yet], that is:

`(y)/(\sin(30))=(18)/(\sin (60))`

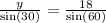
From this we solve for y:

`\Rightarrow y=(18\sin(30))/(\sin(60))\Rightarrow y=6\sqrt[]{3}`

*Second: Now that we have the measure of the other side of the triangle (6sqrt3, approximately 10.4 inches) we find the measure of the largest side:

`h=\sqrt[]{18^2+(6\sqrt[]{3})^2}\Rightarrow h=12\sqrt[]{3}`

So, the length of the longest side is 12sqrt(3) inches (Approximately 20.8 inches).