Question

two buses leave a station at the same time and travel in opposite directions. one bus travels 19 km/h faster than the other. If the two buses are 494 kilometers apart after 2 hours, what is the rate of each bus?

Answer 1

From the scenario, we've been given the following key points:

Given: Let's name first the two buses, Bus A and Bus B.

Bus A = Travels 19 kph faster than Bus B.

Traveling in opposite directions = Two buses are 494 km apart in 2 hours.

From the given, we make this equation:

`\text{ 2x + 2y = 494 km}`

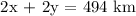
Where,

x = speed of Bus A.

y = speed of Bus B.

2x = distance covered by Bus A after two hours with their constant speed.

2y = distance covered by Bus B after two hours with their constant speed.

But,

Bus A = Travels 19 kph faster than Bus B. We get, x = y + 19

Let's now compute the speed of Bus B.

`\text{ 2x + 2y = 494 }\rightarrow\text{ 2(y + 19) + 2y = 494}`
`\text{ 2y + 38 + 2y = 494 }\rightarrow\text{ 4y = 494 - 38}`
`\text{ 4y = 456 }\rightarrow\text{ y = }(456)/(4)`
`\text{ y = 114 kph}`

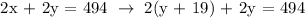
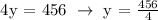
Therefore, the speed of Bus B is 114 km/h or kph.

The speed of Bus A will be = y + 19 = 114 + 19 = 133 km/h or kph.

Speed of Bus A = 133 km/h

Speed of Bus B = 114 km/h