Question

Draw a closed circle on -6 and shade to the left.Draw an open circle on -6 and shade to the right.Draw an open circle on -6 and shade to the left.Draw a closed circle on -6 and shade to the right.

Answer 1

Given:

An inequality is

`(x)/(3)\leq-2`

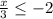
Required:

Draw the graph of the inequality.

Step-by-step explanation:

The given inequality is

`(x)/(3)\leqslant-2`

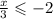
Multiply both sides by 3.

`\begin{gathered} (x)/(3)*3\leqslant-2*3 \\ x\leq-6 \end{gathered}`

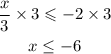
The graph of the inequality is

Thus the solution region to the inequality is the closed point at x = -6 and the region to the left side because the inequality consists of the equal and the less significant.

Final Answer:

The first option is the correct answer.