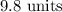Question

Use the distance formula distance, to find the distance to the nearest tenth , between each pair of points A(6,2) and D(-3,-2)

Answer 1

The distance between A and D to the nearest tenth is;

`9.8\text{ units}`

Step-by-step explanation:

Given the two points;

`A(6,2)\text{ and D(-3,-2)}`

Applying the distance between two points formula;

`d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}`

substituting the given coordinates we have;

`\begin{gathered} AD=\sqrt[]{(-3-6)^2+(-2-2)^2} \\ AD=\sqrt[]{(-9)^2+(-4)^2} \\ AD=\sqrt[]{81+16} \\ AD=\sqrt[]{97} \end{gathered}`

Simplifying;

`\begin{gathered} AD=9.8488578 \\ AD\approx9.8 \end{gathered}`

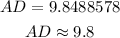
Therefore, the distance between A and D to the nearest tenth is;

`9.8\text{ units}`