Question

I need help with my math

Answer 1

Answer:

y = 2x + 8

Explanations:

The equation of a line passing through the points (x₁, y₁ ) and (x₂, y₂) is given as:

y - y₁ = m (x - x₁)

where m represents the slope of the line, and is calculated by the formula:

`m\text{ = }(y_2-y_1)/(x_2-x_1)`

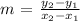
For the line passing through the points (-2, 4) and (1, 10):

x₁ = -2, y₁ = 4, x₂ = 1, y₂ = 10

Calculate the slope:

`\begin{gathered} m\text{ = }(10-4)/(1-(-2)) \\ m\text{ = }(6)/(3) \\ m\text{ = 2} \end{gathered}`

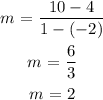
Substitute m = 2, x₁ = -2, and y₁ = 4 into the equation y - y₁ = m (x - x₁)

`\begin{gathered} y\text{ - 4 = 2(x - (-2))} \\ y\text{ - 4 = 2(x + 2)} \\ y\text{ - 4 = 2x + 4} \\ y\text{ = 2x + 4 + 4} \\ y\text{ = 2x + 8} \end{gathered}`

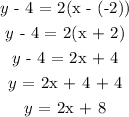
The equation of the line is therefore:

y = 2x + 8