Question

I need help with a geometry question, ill attach it, the cutoff text says the measure of each side

Answer 1

We know that in a triangle WXY,

`\begin{gathered} \angle X\cong\angle Y \\ WX=9x-11 \\ WY=7x-3 \\ XY=4x+1 \end{gathered}`

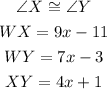
And we want to find the value of x and the length of each side. For doing so, we will do a figure:

And, as the angles X and Y are congruent, the opposite sides to those two angles are also congruent (the sides shown):

Thus:

`WX=WY`

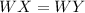
Which means that:

`\begin{gathered} 9x-11=7x-3 \\ \text{And solving for x we obtain:} \\ 9x-11-7x=7x-3-7x \\ 2x-11=-3 \\ 2x-11+11=-3+11 \\ 2x=8 \\ x=(8)/(2)=4 \end{gathered}`

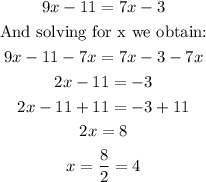
This means that the value of x is 4. Now, we replace the value of x on each expression to find the measure of the sides:

`\begin{gathered} WX=9x-11=9(4)-11=36-11=25=WY \\ XY=4x+1=4(4)+1=16+1=17 \end{gathered}`

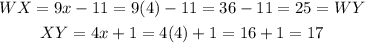
This means that WX=WY=25 and XY=17.