ANSWER
x² + y² = 9
Step-by-step explanation
The equation of a circle in standard form is,

Where (h, k) is the center of the circle and r is the radius.
In this case, the center is (0, 0), so the equation is,
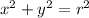
We do not know the radius of the circle, but we know that it passes through (-3, 0), so we can replace x and y with the coordinates of this point to find r,
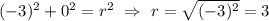
Hence, the equation of the circle is x² + y² = 9.