Question

(b) If the ring's inner circumference can vary between 5.5 centimeters and 6.5 centimeters, how can the radius vary? (Round your answers to four decimal places.)

Answer 1

This question relates to the circumference of a circle which is given as

Circumference of a Circle.

The circumference of a circle is given as

`\begin{gathered} C=2\pi r \\ \pi=3.14 \\ r=\text{radius} \end{gathered}`

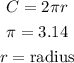
Using this formula, we can solve for the radius of the circle.

We were given the circumference of the circle as 6cm, let us substitute the values and solve for it.

`\begin{gathered} c=2\pi r \\ 6=2\pi r \\ 6=2*3.14* r \\ 6=6.28r \\ r=(6)/(6.28) \\ r=0.955 \end{gathered}`

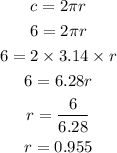
The radius of the inner circumference is 0.955cm.

Assuming the inner circumference varies between 5.5cm to 6.5cm, the radius will varies as

Applying the same formula as we have used above

And when the circumference is at 6.5cm

When the circumference varies between 5.5cm to 6.5cm, the radius also varies from 0.87cm to 1.04cm.