Question

A 500 kg object is hanging from a spring attached to the ceiling. If the spring constant in the spring is 900 N/kg, how far does the spring stretch?

Answer 1

We are given that a 500 kg object is hanging from a spring. To determine the amount the spring is stretched we will use Hook's law, which states the following:

`F=kx`

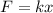
Where:

`\begin{gathered} F=\text{ force} \\ k=\text{ spring constant} \\ x=\text{ distance stretched} \end{gathered}`

Since the object is hanging the only force acting on the spring is the weight of the object. The weight of the object is:

`F_g=mg`

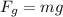
Where:

`\begin{gathered} m=\text{ mass} \\ g=\text{ acceleration of gravity} \end{gathered}`

Plugging in the values we get:

Solving the operations:

`F_g=4900N`

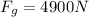
Now we solve for "x" from Hook's law by dividing both sides by "k":

`(F)/(k)=x`

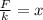
Now we plug in the known values:

`(4900N)/(900(N)/(m))=x`

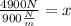
Solving the operations:

`5.4m=x`

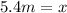
Therefore, the spring is stretched by 5.4 meters.